Blog >
Black Scholes Model: What is it, Formula, Calculator
Black Scholes Model: What is it, Formula, Calculator
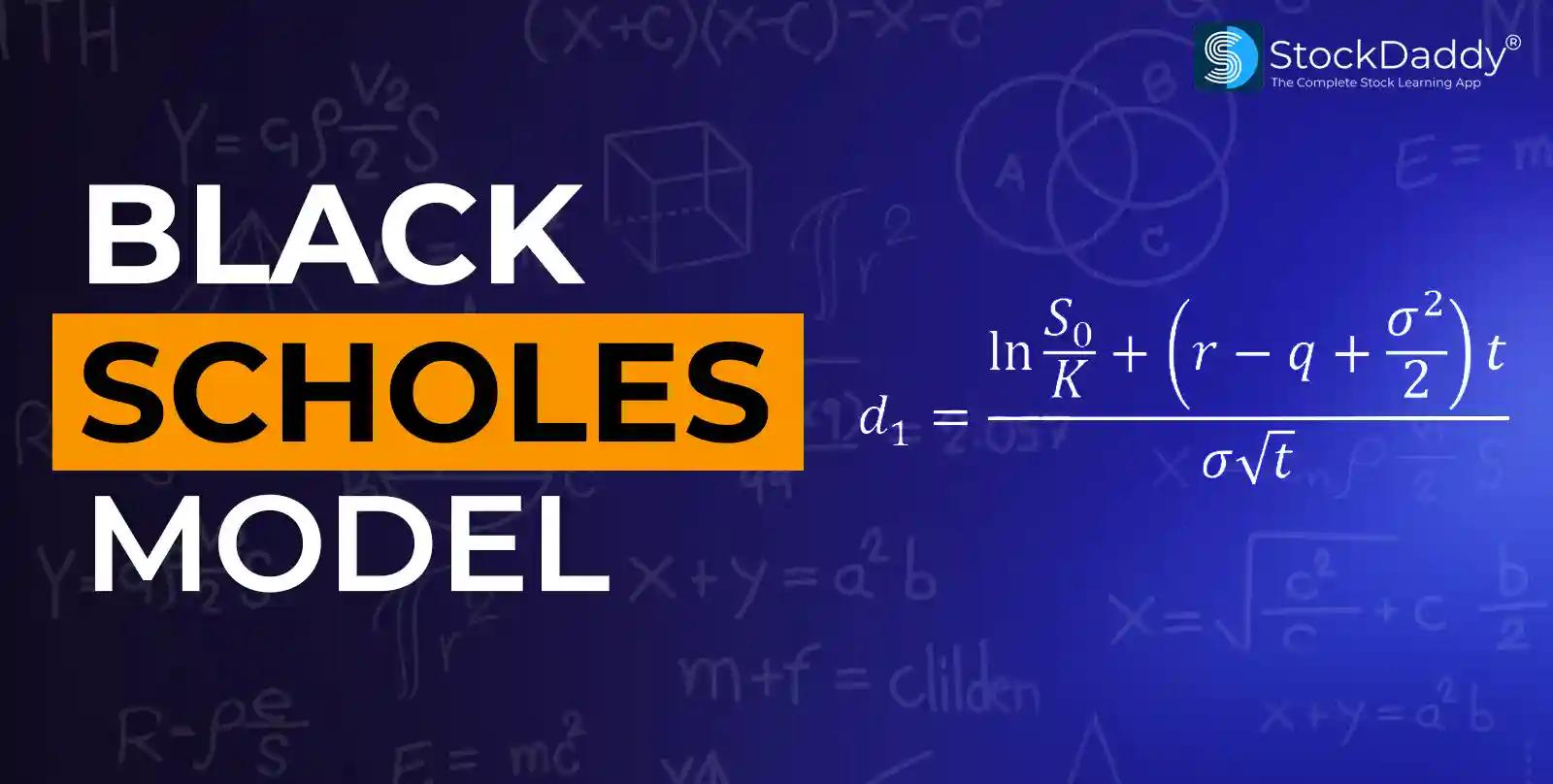
What is Black Scholes Model?
The Black-Scholes option pricing model functions as a kind of specialized calculator for calculating how much money should be paid or received when buying or selling something called "options." These options function similarly to future commitments to buy or sell something at a specific price.
What makes this calculator so crucial? It enables people to assess the value of these promises. Consider saying you'll purchase someone a toy in the future. The toy will cost more if it becomes very popular, right? It should therefore be worth more for you to agree to buy it. You can determine how much more with the calculator.
This calculator was designed on two main concepts:
-
Hedging: Hedging is similar to balancing out a situation. You risk losing money if you make a purchase commitment but the item's price rises. However, the calculator explains how to balance that risk so you don't suffer a severe loss.
-
Partial Differential Equations: Partial differential equations are a type of fancy math that the calculator utilizes to ensure fairness. To ensure that everyone agrees on the pricing is like performing a magic trick.
So, to put it simply, the Black-Scholes model assists people in determining how much they should pay or receive for options while ensuring fairness through sophisticated arithmetic and balancing techniques.
Importance of Black Scholes Model in Options Pricing
The Black-Scholes Model is a crucial component of finance. It aids in determining how much a particular option ought to cost. Like a special offer, an option allows you to buy or sell anything at a specific price in the future.
This model uses a few crucial figures to inform us of the option's cost. These figures include the cost of the item we wish to buy or sell (such as a stock), the option's special pricing, the amount of time left before the offer expires, the amount the item's price fluctuates, and the amount of money you can deposit safely in the bank.
Prior to the development of this model, people made pricing decisions based on sentiments and educated assumptions. Because prices weren't always equitable or uniform for everyone, this led to issues.
However, the Black-Scholes Model made a difference. It enabled us to determine a reasonable price for our selections. This improved the efficiency of the market and made it simpler for individuals to trade options.
We learned about controlling risk in finance thanks to the model. Risk means that if something goes wrong, you could occasionally lose money. When we make financial bargains, the Black-Scholes Model teaches us how to prevent ourselves from suffering excessive financial losses. The financial market is now safer as a result.
So, to put it simply, the Black-Scholes Model is a clever instrument that makes it simpler to understand how much options should cost and aids in our financial security. In the world of finance, it's huge!
Black Scholes Model Assumptions
The Black-Scholes Model can be compared to a recipe for determining the value of various financial assets. To employ this formula, however, we must rely on some educated estimates or "assumptions."
-
European Options: This model only works for a certain type of option called "European." Imagine you have a special coupon that you can only use on a specific date. That's like a European option. But, in real life, some coupons can be used anytime. This model doesn't work for those.
-
No Dividends: The model assumes that the thing we're talking about (let's say, a stock) doesn't give you any extra money while you're waiting. In real life, some stocks do pay you a little extra along the way, like a bonus. This model doesn't consider that.
-
Efficient Market Idea: It's like saying that everyone knows everything about this stock, and the price already has all the information in it. So, you can't find a way to make extra money because it's like a super smart market. In real life, markets aren't always super smart.
-
Risk-Free Interest Rate: The model thinks the interest rate is always the same and won't change. But in real life, it can go up and down, and that affects how much things are worth.
-
Lognormal Thing: This is a fancy way of saying the model thinks that the prices of things go up and down in a particular way. But in real life, they might not always follow this exact pattern.
-
Constant Volatility: The model assumes that how much prices move up and down stays the same. But in real life, it can change a lot. Imagine a rollercoaster that sometimes goes super fast and sometimes slow – that's like how prices move in real life.
Components of Black Scholes Formula
The Greek Letters help us comprehend how special contracts vary as elements like the price and time change, and the Black-Scholes Formula functions as a tool to determine how much they should cost. Making informed decisions about buying and selling goods is akin to math wizardry.
- Call Option Pricing: This part figures out how much a special contract costs. The contract lets you buy something at a set price.
- Put Option Pricing: This part figures out how much another special contract costs. This contract lets you sell something at a set price.
- Greek Letters: These are not really letters from Greece! They help us understand how the special contracts change when other things change.
- Delta: Delta tells us how much the special contract's price changes when the thing you want to buy or sell changes in price.
- Gamma: Gamma tells us how fast Delta changes when the thing you want to buy or sell changes in price.
- Vega: Vega tells us how the special contract's price changes when the thing you want to buy or sell becomes more or less shaky.
- Theta: Theta tells us how fast the special contract's price changes as time goes by.
- Rho: Rho tells us how the special contract's price changes when the interest rate without risk changes.
Practical Application of the Black Scholes Model
The Black-Scholes Model is a useful tool in various areas:
-
Financial Markets: People in finance use the Black-Scholes model a lot. It helps figure out how much to pay for options, which are like special deals for buying and selling things. This helps them decide where to invest their money and how to stay safe with their investments.
-
Corporate Finance: Big companies use the model to decide how much their workers should get when they offer them a chance to buy company shares. It helps them make fair choices about pay.
-
Risk Management: People who invest money and big banks use the Black-Scholes model to understand and handle risks. It tells them how different things can affect the prices of options, which helps them keep their money safe.
-
Algorithmic Trading: Some smart computer programs use the Black-Scholes model to find deals in the stock market. They look for options that might not have the right price and try to make money from those situations.
The Black-Scholes Model is essentially a specialized calculator that assists those working in finance with a variety of tasks, including determining how much to pay for options and avoiding excessive losses. In the world of finance and investing, it is quite significant.
Limitations of Black Scholes Model
-
Problems with the Model's Ideas: Certain concepts employed by the Black-Scholes model don't always hold true in practice. When we try to calculate how much options are worth and how to control the risks, this can cause it to provide incorrect answers.
-
Wrong Guesses About How Things Change: The model presumes that things change steadily, but occasionally they alter dramatically and unexpectedly. As a result, the model may give us incorrect information regarding the cost of alternatives and risk mitigation strategies.
-
Can't Handle Really Surprising Events: According to the Black-Scholes model, price changes have a predictable pattern. But occasionally, truly unexpected occurrences take place that the model does not anticipate. As a result, the forecasts of the model may differ greatly from what actually occurs in the market.
Therefore, to put it simply, the Black-Scholes model is like a tool that occasionally works well but isn't flawless. When we strive to understand options and how to stay secure in the financial world, it could give us the incorrect answers.
Alternatives to Black Scholes Model
There are some other ways people use to figure out how to buy or sell things, and they're not like the Black-Scholes model. Let us explain them in simpler terms:
-
Binomial Tree Model: Imagine that you are interested in the price of something in the future. This approach creates a type of tree with various cost estimations. It states that the current price may increase or decrease. The model may estimate how much something might be valued in the future by estimating how probable each of these events is to occur.
-
Trinomial Tree Model: Compared to the binomial tree, this has an additional possibility. To the "go up" and "go down" options, "no change" is added. This is more true because circumstances can occasionally remain unchanged.
-
Stochastic Volatility Models: The Black-Scholes model makes the assumption that the volatility, or degree of market turbulence, remains constant. But in reality, it is different. By estimating how much things might fluctuate based on certain unpredictability, these models attempt to account for this.
-
Jump-Diffusion Models: These models are even more luxurious. They take into account both tiny, gradual price shifts and abrupt, significant ones. The equivalent would be to state, "Sometimes, things change a little bit every day, but other times, they can suddenly jump a lot in one go." These models aid in our understanding of the sometimes highly erratic pricing found in markets.
These thus represent various approaches to comprehending potential price changes in the future. They aid anyone looking to buy or sell products in making wiser choices, particularly when prices are subject to sudden changes. Each model has its own advantages and may be applied to various circumstances.
Conclusion
The Black-Scholes model, to put it simply, is a crucial tool in the world of finance and investments. People continue to use it frequently despite its flaws since it is simple to comprehend and widely accepted.
We utilize this model to make investment and risk management decisions as well as to determine how much to pay for options.
Experts will undoubtedly work to improve this model in the future. They'll strive to improve the model's predictions and incorporate elements like rapid changes in prices and how prices fluctuate in unexpected ways.
We can improve our models for determining option pricing and make them more practical in the real world of finance by conducting this research.
